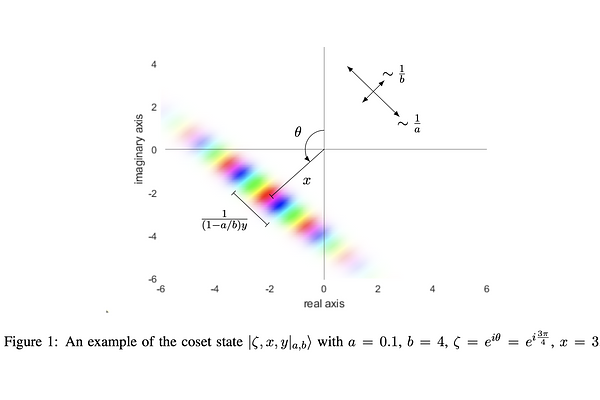
Eric Culf, Thomas Vidick, Victor V. Albert

We develop an extension of a recently introduced subspace coset state monogamy-of-entanglement game [Coladangelo, Liu, Liu, and Zhandry; Crypto'21] to general group coset states, which are uniform superpositions over elements of a subgroup to which has been applied a group-theoretic generalization of the quantum one-time pad. We give a general bound on the winning probability of a monogamy game constructed from subgroup coset states that applies to a wide range of finite and infinite groups. To study the infinite-group case, we use and further develop a measure-theoretic formalism that allows us to express continuous-variable measurements as operator-valued generalizations of probability measures. We apply the monogamy game bound to various physically relevant groups, yielding realizations of the game in continuous-variable modes as well as in rotational states of a polyatomic molecule. We obtain explicit strong bounds in the case of specific group-space and subgroup combinations. As an application, we provide the first proof of one sided-device independent security of a squeezed-state continuous-variable quantum key distribution protocol against general coherent attacks.