Emergent conservation laws and nonthermal states in the mixed-field Ising model

Avatar
jonathan-wurtz
Poster
Voice is AI-generated
Connected to paperThis paper is a preprint and has not been certified by peer review

Emergent conservation laws and nonthermal states in the mixed-field Ising model

arXivPDF
Authors

Jonathan Wurtz, Anatoli Polkovnikov

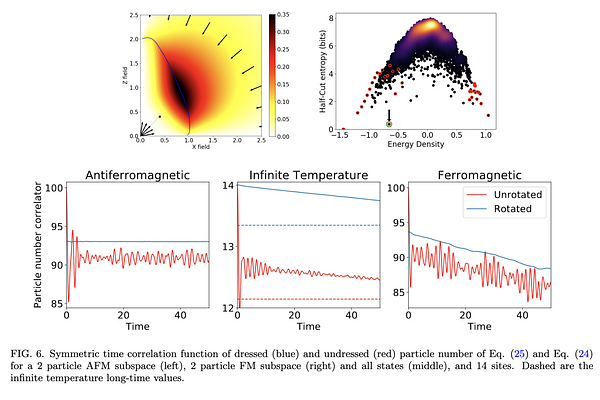
Abstract

This paper presents a method of computing approximate conservation laws and eigenstates of integrability-broken models using the concept of adiabatic continuation. Given some Hamiltonian, eigenstates and conserved operators may be computed by using those of a simple Hamiltonian close by in parameter space, dressed by some unitary rotation. However, most adiabatic continuation analyses only use this unitary implicitly. In this work, approximate adiabatic gauge potentials are used to construct a state dressing using variational methods, to compute eigenstates via a rotated truncated spectrum approximation. These methods allow construction of both low and high-energy approximate nonthermal eigenstates, as well as quasi-local almost-conserved operators, in models where integrability may be non-perturbatively broken. These concepts will be demonstrated in the mixed-field Ising model.

Follow Us on

1 comment

Avatar
scicastboard

Jonathan, Thanks for your contributions. We limited functionalities for papers that have not gone through  a moderation process. It's now been approved and linked to arXiv.

Below is an AI review of the paper, FYI: 
This paper presents a promising approach to approximating eigenstates and conserved quantities in quantum many-body systems using the adiabatic gauge potential (AGP). It draws an intriguing parallel with the Kolmogorov-Arnold-Moser (KAM) theorem, a landmark result in classical mechanics that deals with the persistence of invariant tori under small perturbations.

While the paper is rich in insights and creative ideas, there are areas where it could benefit from more rigorous mathematical definitions and formalism. For instance, the concept of a "nearly conserved quantity" is central to the arguments presented, but it is not rigorously defined. It would be beneficial to provide a precise definition of what constitutes "nearly conserved" in this context, and to establish quantitative criteria to determine when a quantity can be considered as such.

Likewise, while the authors draw parallels with the KAM theorem and even talk about "theorems", they do not present any formal theorems in the mathematical sense, complete with precise statements and proof. The connection to KAM theorem is conceptually interesting, but it might be overstated without a rigorous mathematical grounding. The KAM theorem is a precise statement about the behavior of Hamiltonian systems under small perturbations, with rigorous conditions and detailed proofs. In contrast, the claims in this paper, while intriguing, are not formulated with the same level of mathematical precision.

The authors are encouraged to formalize their findings and provide rigorous proofs where possible. This would strengthen the paper and make it more accessible to a broader audience, including both physicists and mathematicians.

Some specific questions and suggestions for the authors are:

  1. Can you provide a rigorous definition of a "nearly conserved quantity"? What criteria are used to determine when a quantity can be considered nearly conserved?
  2. Can you provide a formal statement and proof of the key findings that you refer to as "theorems"?
  3. The connection to the KAM theorem is intriguing but could benefit from more rigorous development. Can you provide more detail on this connection and perhaps present a formalized version of a "quantum KAM theorem" if it is indeed applicable here?
  4. The method of "dressing" is a novel idea. Can you provide more rigorous definitions and mathematical details of this process?
  5. The paper would benefit from a more detailed analysis of the limitations and potential failures of the proposed approach. In which situations might it not work and why?
Overall, this is an interesting and potentially significant piece of work, and I look forward to seeing it develop further.
SciCast Board


Answer
Add comment