Oluwadara Ogunkoya, Joonho Kim, Bo Peng, A. Barış Özgüler, Yuri Alexeev

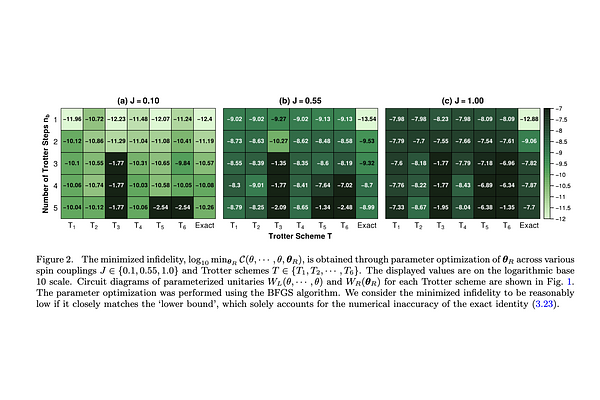
Quantum information processing has witnessed significant advancements through the application of qubit-based techniques within universal gate sets. Recently, exploration beyond the qubit paradigm to $d$-dimensional quantum units or qudits has opened new avenues for improving computational efficiency. This paper delves into the qudit-based approach, particularly addressing the challenges presented in the high-fidelity implementation of qudit-based circuits due to increased complexity. As an innovative approach towards enhancing qudit circuit fidelity, we explore algebraic relations, such as the Yang-Baxter-like turnover equation, that may enable circuit compression and optimization. The paper introduces the turnover relation for the three-qutrit time propagator and its potential use in reducing circuit depth. We further investigate whether this relation can be generalized for higher-dimensional quantum circuits, including a focused study on the one-dimensional spin-1 Heisenberg model. Our work outlines both rigorous and numerically efficient approaches to potentially achieve this generalization, providing a foundation for further explorations in the field of qudit-based quantum computing.