Alexis Coissard, Adolfo G. Grushin, Cécile Repellin, Louis Veyrat, Kenji Watanabe, Takashi Taniguchi, Frédéric Gay, Hervé Courtois, Hermann Sellier, Benjamin Sacépé

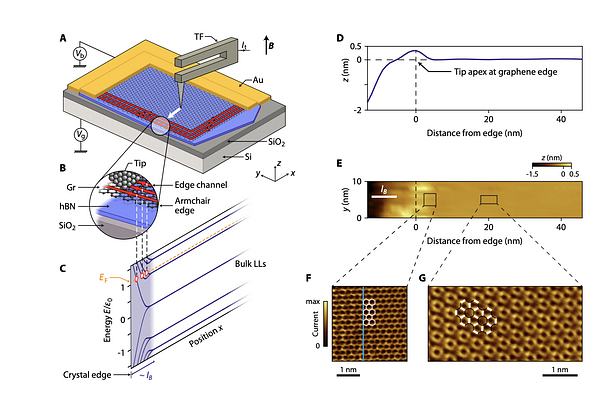
Electronic edge states in topological insulators have become a major paradigm in physics. The oldest and primary example is that of quantum Hall (QH) edge channels that propagate along the periphery of two-dimensional electron gases (2DEGs) under perpendicular magnetic field. Yet, despite 40 years of intensive studies using a variety of transport and scanning probe techniques, imaging the real-space structure of QH edge channels has proven difficult, mainly due to the buried nature of most 2DEGs in semiconductors. Here, we show that QH edge states in graphene are confined to a few magnetic lengths at the crystal edges by performing scanning tunneling spectroscopy up to the edge of a graphene flake on hexagonal boron nitride. These findings indicate that QH edge states are defined by boundary conditions of vanishing electronic wavefunctions at the crystal edges, resulting in ideal one-dimensional chiral channels, free of electrostatic reconstruction. We further evidence a uniform charge carrier density at the edges, contrasting with conjectures on the existence of non-topological upstream modes. The absence of electrostatic reconstruction of quantum Hall edge states has profound implications for the universality of electron and heat transport experiments in graphene-based systems and other 2D crystalline materials.