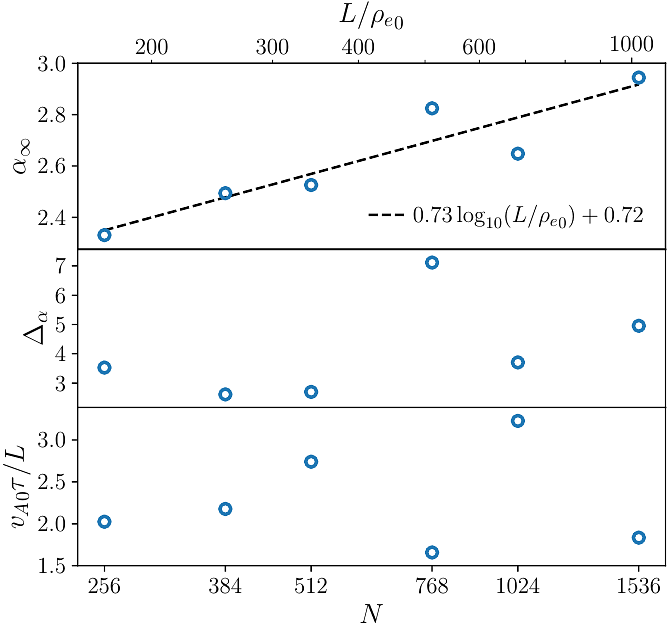
Kai W. Wong Univ. Colorado Boulder, Vladimir Zhdankin Univ.Wisconsin-Madison Center for Computational Astrophysics, Flatiron Inst Princeton Univ, Dmitri A. Uzdensky Univ. Colorado Boulder Univ. Oxford, Gregory R. Werner Univ. Colorado Boulder, Mitchell C. Begelman Univ. Colorado Boulder JILA, Univ. Colorado & NIST

Turbulent, relativistic nonthermal plasmas are ubiquitous in high-energy astrophysical systems, as inferred from broadband nonthermal emission spectra. The underlying turbulent nonthermal particle acceleration (NTPA) processes have traditionally been modelled with a Fokker-Planck (FP) diffusion-advection equation for the particle energy distribution. We test FP-type NTPA theories by performing and analysing particle-in-cell (PIC) simulations of turbulence in collisionless relativistic pair plasma. By tracking large numbers of particles in simulations with different initial magnetisation and system size, we first test and confirm the applicability of the FP framework. We then measure the FP energy diffusion ($D$) and advection ($A$) coefficients as functions of particle energy $\gamma m c^2$, and compare their dependence to theoretical predictions. At high energies, we robustly find $D \sim \gamma^2$ for all cases. Hence, we fit $D = D_0 \gamma^2$ and find a scaling consistent with $D_0 \sim \sigma^{3/2}$ at low instantaneous magnetisation $\sigma(t)$, flattening to $D_0 \sim \sigma$ at higher $\sigma \sim 1$. We also find that the power-law index $\alpha(t)$ of the particle energy distribution converges exponentially in time. We build and test an analytic model connecting the FP coefficients and $\alpha(t)$, predicting $A(\gamma) \sim \gamma \log \gamma$. We confirm this functional form in our measurements of $A(\gamma,t)$, which allows us to predict $\alpha(t)$ through the model relations. Our results suggest that the basic second-order Fermi acceleration model, which predicts $D_0 \sim \sigma$, may not be a complete description of NTPA in turbulent plasmas. These findings encourage further application of tracked particles and FP coefficients as a diagnostic in kinetic simulations of various astrophysically relevant plasma processes like collisionless shocks and magnetic reconnection.