Christian Faßbender, Tim Bürchner, Philipp Kopp, Ernst Rank, Stefan Kollmannsberger

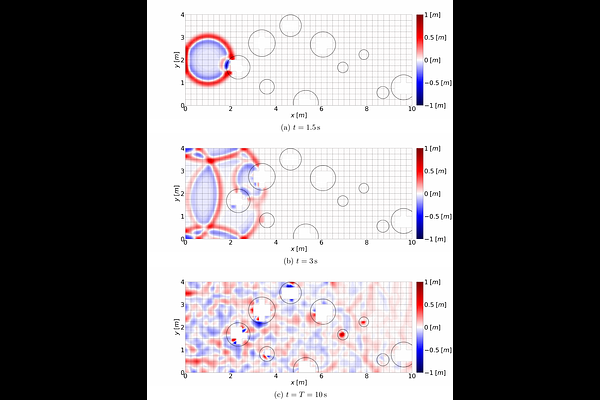
Immersed boundary methods simplify mesh generation by embedding the domain of interest into an extended domain that is easy to mesh, introducing the challenge of dealing with cells that intersect the domain boundary. Combined with explicit time integration schemes, the finite cell method introduces a lower bound for the critical time step size. Explicit transient analyses commonly use the spectral element method due to its natural way of obtaining diagonal mass matrices through nodal lumping. Its combination with the finite cell method is called the spectral cell method. Unfortunately, a direct application of nodal lumping in the spectral cell method is impossible due to the special quadrature necessary to treat the discontinuous integrand inside the cut cells. We analyze an implicit-explicit (IMEX) time integration method to exploit the advantages of the nodal lumping scheme for uncut cells on one side and the unconditional stability of implicit time integration schemes for cut cells on the other. In this hybrid, immersed Newmark IMEX approach, we use explicit second-order central differences to integrate the uncut degrees of freedom that lead to a diagonal block in the mass matrix and an implicit trapezoidal Newmark method to integrate the remaining degrees of freedom (those supported by at least one cut cell). The immersed Newmark IMEX approach preserves the high-order convergence rates and the geometric flexibility of the finite cell method. We analyze a simple system of spring-coupled masses to highlight some of the essential characteristics of Newmark IMEX time integration. We then solve the scalar wave equation on two- and three-dimensional examples with significant geometric complexity to show that our approach is more efficient than state-of-the-art time integration schemes when comparing accuracy and runtime.