Soumya Sengupta, Manika Singla, Fikret Anli

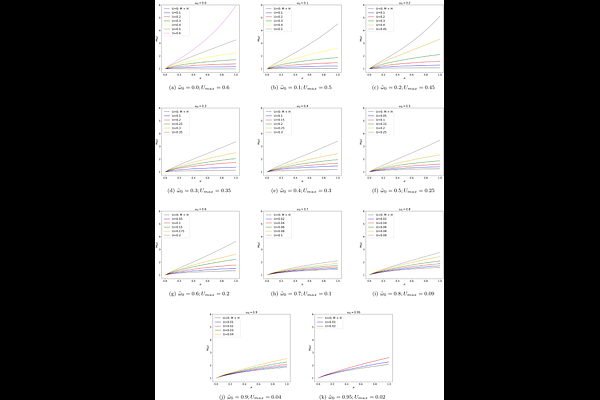
Chandrasekhars $H(\mu)$-function has long been a cornerstone of radiative transfer theory in semi-infinite, isotropically scattering atmospheres subjected to external illumination. However, this classical formulation does not account for thermal emission arising from internal heat sources, which is critical in a variety of astrophysical contexts such as hot Jupiters, brown dwarfs, and irradiated exoplanets, where the re-radiation of absorbed stellar energy significantly alters the emergent intensity. To address this limitation, we extend the diffuse reflection framework to incorporate both isotropic scattering and intrinsic thermal emission, leading to a generalized function known as the $M(\mu)$-function. Building on the formalism of Chandrashekhar (1960) combined with the recent work by Sengupta(2021), we derive the governing integral equations for the $M$-function and express it in terms of three key physical parameters: the radiation direction cosine $\mu$, the thermal emission coefficient $U(T) = B(T)/F$, and the single scattering albedo $\tilde{\omega}_0$. We implement a numerically stable iterative scheme, using Gaussian quadrature method to compute high-precision values of $M(\mu, U, \tilde{\omega}_0)$ across a broad parameter space. In the zero-emission limit, our results recover the classical $H$-function, thus validating the method.We provide comprehensive values of $M$-function for $\mu \in [0, 1]$, $U < 0.7$, and $\tilde{\omega}_0 < 1$, enabling direct application to modeling diffusely reflected intensities from thermally emitting atmospheres.As a case study, we apply this formalism to exoplanet K2-137b and identify the wavelength range (0.85 -2.5 $\mu$m) where the model is most applicable, highlighting its relevance to the observation range of the telescopes JWST, HST, ARIEL.